Abstract

A dimmer is an electronic device that controls alternate voltage applied to a lamp through delivering a selected portion of the mains sinusoid. Engineer, designing a dimmer needs to estimate how big this portion should be to get a desired luminance level. This article uses a model of incandescent lamp, tungsten resistivity, and human eye spectral efficiency to derive dependency of produced luminous flux over the voltage, gives a simple analytical function that describes this dependency with good accuracy (±2% comparing to the model).

Download PDF version of this article

Structured Abstract

Purpose	To discover a dependency of luminous flux produced by an incandescent lamp on the applied voltage
Methodology/Approach	Numerical analysis on a theoretical model of an incandescent lamp
Findings	Analytical function for approximate estimating of luminous flux for the applied voltage with ±2% accuracy
Research limitations/implications	Model does not encounter thermal conduction and convection in the lamp.
Practical implications (if applicable)	Provided function has some degree of dependency on the lamp’s design (namely, nominal filament temperature)

Definitions

Process of controlling some output value (such as voltage U or luminous flux L) we will denote as a control function , defined on the control parameter :

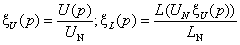(1)

Each of  monotonously increase on the defined range. Control functions defined on a different (other than ) argument we will denote in this article as .

Commonly used are linear () and logarithmic () control functions. To implement a desired control function , we needs to know what is  dependency. 
The following chapters give an attempt to derive  using a model suggested in (Agraval 1996).

Incandescent Lamp Model

An incandescent lamp is characterized with few nominal values: P_N – power consumption, U_N – nominal voltage, L_N – nominal luminous flux, produced by the lamp at nominal voltage. The flux is produced by filament, incandesced to nominal working temperature T_N.
When lower voltage U<U_N is applied, operating temperature T of filament is lower T_N and therefore it produces less luminosity L.

Filament Resistance

Power, consumed by a lamp, is expressed with Ohm low:

(2)

where  defines dependency of filament resistance over the temperature relatively to its nominal resistance R_N, which can be evaluated via P_N and U_N:

(3)

Dependency  for tungsten is not linear in the working range of temperatures (1000-2500K). For this model we will use polynomial approximation of tungsten resistance ρ, [Harang 2003]:

(4)

 can be expressed via  as the following:

(5)

Filament Temperature

During operation, filament radiates electromagnetic energy and dissipates heat via conduction and convection. To estimate radiation, filament is modeled [Agraval 1996] as a simple non-ideal blackbody that obeys Plank’s radiation law:

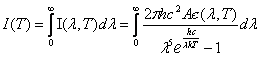(6)

where  is power radiated between wavelength  and ,  – tungsten emittance, and A is filament area. The total power emitted over all wavelengths is:

(7)

where σ is the Stefan-Boltzman constant and  is average emittance over all wavelengths, which is approximated as a second order polynomial (Harang 2003):

(8)

In a steady-state operation, power applied to the lamp is in balance with power radiated and dissipated to outside ambient. Dissipation has two constituents – conduction and convection. Agraval in [Agraval 1996] has suggested a reasonable way of accounting dissipation as a factor of input power:

(9)

Solving (8) for P:

(10)

Substituting left side of (10) with right side of (2):

(11)

Using (11), we may derive :

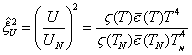(12)

Figure 1 illustrates dependency

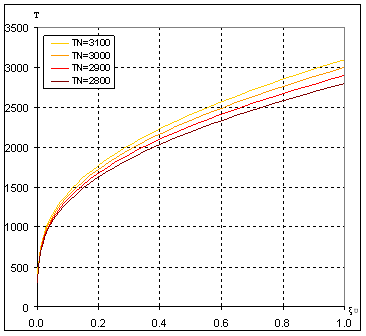
Figure 1. Dependency of T on fraction of applied voltage ξ_U for different T_N

Luminance

Not all power radiated by filament has its effect on luminous flux. Some of the energy is absorbed by bulb glass and dissipated as heat. Although, this absorption depends on λ, we will simplify this absorption to a constant factor η<1.

Major part of the emitted energy is not visible to human eye. This is described as spectral efficiency function S(λ), approximated as the following [Agraval 1996]:

(13)

Thus, total luminous flux produced by a lamp can be defined as:

(14)

where

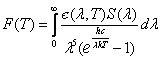(15)

Thereby, control function  can be expressed as a function of T:

(16)

For working range of temperatures and visible area, emissivity є(λ,T) can be approximated as the following [Larrabee 1957]:

(17)

where T is in °K and λ in nm. Substituting (17) in (16) and integrating numerically (16) we get dependency, shown on Figure 2:

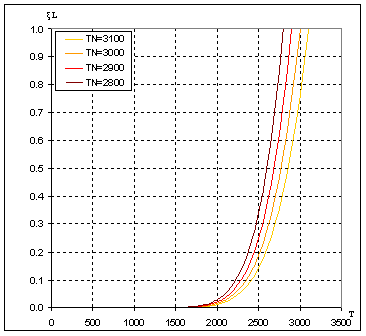
Figure 2. Dependency ξ_L(T) for different T_N

Solving Luminance vs. Voltage

Using T as a parametric variable, we may numerically compute (11), (15) and graphically solve dependency ξ_L(ξ_U), as shown on Figure 3:

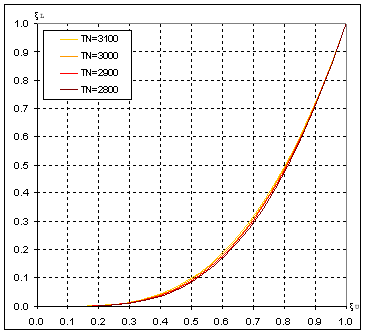
Figure 3. Dependency ξ_L(ξ_U) for different T_N

As Figure 3 indicates, ξ_L(ξ_U) is affected by a design factor – nominal temperature T_N.

Approximation

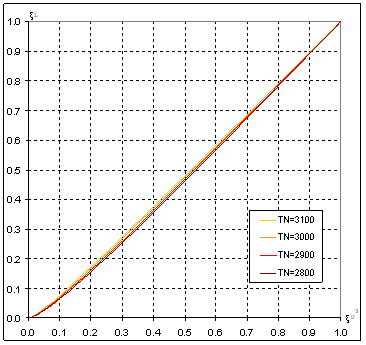
Figure 4. Dependency ξ_L(ξ_U³)

As it appears (see Figure 4), dependency ξ_L(ξ_U³) looks quite linear for ξ_U³>0.2 and has some higher-order dependency for ξ_U³<0.2. Therefore we will try approximating ξ_L(ξ_U) as two polynoms:

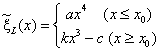(18)

Value ξ_Lat x=1 is equal to 1 by nature of ξ, therefore c can be expressed via k:

(19)

To solve a and x₀ we will require continuality of  and its derived function , this gives us two equations with three unknowns:

(20)

Solving (20) for a and x₀ we get them expressed via k:

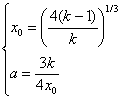(21)

Thereby,  depends on a single constant k, which is then wiggled around to find a value giving lowest RMS deviation . Table 1 lists selected values for few most common nominal temperatures T_N, Figure 5 illustrates this dependency, and Figure 6 shows modeled curves and values, approximated with (20).

Table 1. k values for some T_N

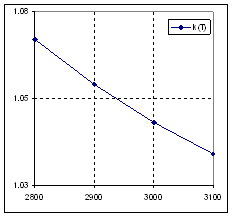
Figure 5. k variations over T_N

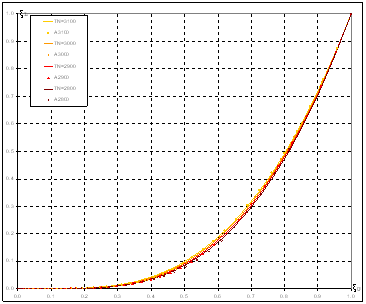
Figure 6. Modeled curves and approximated values (marks)

Since designer of a dimmer may not exactly know nominal temperature of the dimmed lamp, kcan be selected for an average nominal temperature. Suggested value k=1.05 gives ±2% error (against the model) for T_N in range 2800-3100 °K. For this k, equation (20) becomes practically concrete:

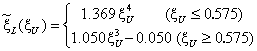(22)

Sine Wave Dimmer

A sine wave dimmer implemented with pulse-width modulation controls applied voltage with a cycle duty, which we will denote as control function ξ_t. Modulation frequency is usually chosen much higher than mains frequency. Therefore, RMS of output voltage is proportional to cycle duty, and control function ξ_U as simple as:

(23)

To make ξ_L(p), linear on parameter p, function ξ_t(p) should be defined as the following:

(24)

Applying (24) to equation (22) we get

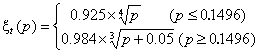(25)

Phase Control Dimmer

When implementing an AC dimmer based on phase control technique, one needs to tabulate cycle duty values ti as a function of desired luminance level pi. 
Following the same approach, we introduce control function ξ_t:

(26)

where t is cycle duty (time when the switch is on) and tM is the mains half period, and f is the mains frequency. Average power  applied to the lamp, can be evaluated as the following:

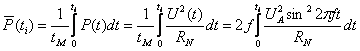(27)

where UA is voltage amplitude. Integrating (27) we can define ξ_P as the following

(28)

Considering that , and substituting (28) to (22) we may estimate .

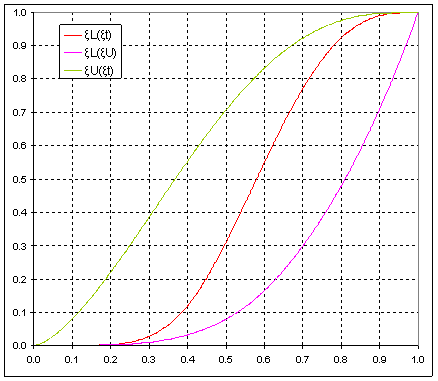
Figure 7. Dimmer control functions ξ_L and ξ_U

Applying approach used in (24) function ξ_t(p) is defined as following:

(29)

Although, reverse function of (28) is not solvable analytically, it can be solved numerically.

Conclusions

As a result of numerical modeling, the following approximation functions are suggested:

• Estimation of produced luminous flux over the voltage:

L = L0 * ((U/U0 < 0.575) ? 1.369 * (U/U0) ^ 4 : (1.05 * (U/U0) ^ 3 - 0.05);

• Tabulation of cycle duty over the control parameter p

t = (p < 0.1496) ? (0.925 * (p) ^ -4) : (0.984 * (p + 0.05) ^ -3);

References

Agraval D.C., Leff H.S., Monon V.J. (1996)
"Efficiency and efficacy of incandescent lamps"
American Journal of Physics, May 1996, Volume 64, Issue 5, pp. 649-654
http://scitation.aip.org/getabs/servlet/GetabsServlet?prog=normal&id=AJPIAS000064000005000649000001&idtype=cvips&gifs=yes

Harang O., Kosch M. J. (2003) "Absolute Optical Calibration Using a Simple Tungsten
Bulb"
Sodankylä Geophysical Observatory Publications, 2003, pp. 92:121-123
http://spaceweb.oulu.fi/28AM/proc_papers/27_harang_et_al_calibration_with_tungsten_bulb.pdf

Larrabee R.D. (1957) "The spectral emissivity and optical properties of tungsten"
Research Laboratory Of Electronics, Massachusetts Institute of Technology
http://dspace.mit.edu/bitstream/1721.1/4755/1/RLE-TR-328-04734719.pdf